The mortgage constant, also known as the loan constant, the debt constant, or the mortgage capitalization rate, is an important concept to understand in commercial real estate finance. Yet, it’s commonly misunderstood. In this article, we’ll take a closer look at the mortgage constant, discuss how it can be used, and then tie it all together with a relevant example.
What is The Mortgage Constant?
First, what exactly is the mortgage constant? The mortgage constant, also known as the loan constant or the debt constant, is defined as annual debt service divided by the loan amount. Here is the formula for the mortgage constant:

In other words, the mortgage constant is the annual debt service amount per dollar of loan, and it includes both principal and interest payments.
How to Calculate the Mortgage Constant
There are two commonly used methods to calculate the mortgage constant. The first simply divides annual debt service by the total loan amount. The second allows you to calculate the mortgage constant for any loan amount by solving for the payment based on a loan amount of $1. Let’s take a look at both methods.
Suppose we have a $1,000,000 loan based on a 6% interest rate and a 20-year amortization. With this information, you can simply find the annual debt service using the above assumptions, then divide the annual debt service by the loan amount.
On our financial calculator, if we plug in 240 months for N, -$1,000,000 for PV, .50% for I (6%/12), and 0 for FV, then we can solve for the monthly payment. To convert this to an annual payment amount, we simply multiply by 12. Note that when you carry out the decimal 2 places you get a monthly payment of $7,164.31, which equals $85,971.73 when multiplied by 12.
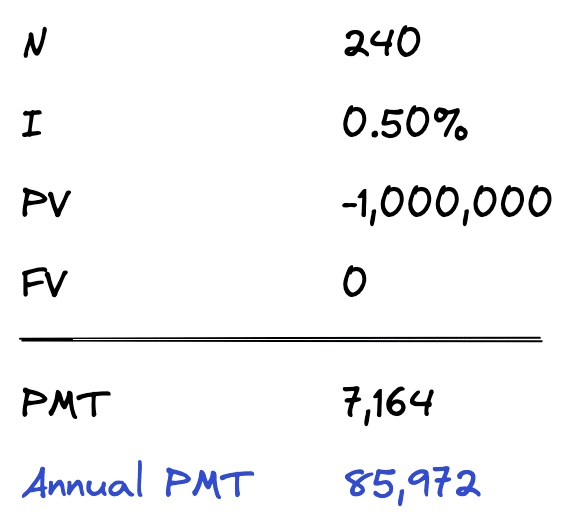
Since the mortgage constant is simply the ratio of annual debt service to the total loan amount, this calculation is just a simple division. In this case, we take $85,972 / $1,000,000 to get a mortgage constant of 0.085972. As a percentage, this would be 8.5972%.
The method above works if you already know the loan amount, but what if you want to find the mortgage constant for any loan amount? If you only know the amortization period and the interest rate, then you can easily solve for the mortgage constant.
This is accomplished by plugging this information into a financial calculator, while using $1 as the present value. For example, consider the same loan terms above of a 20-year amortization (240 months) and a 6% interest rate (0.50% per month). Since we don’t know what the loan amount is (present value), we can use $1 as the present value:
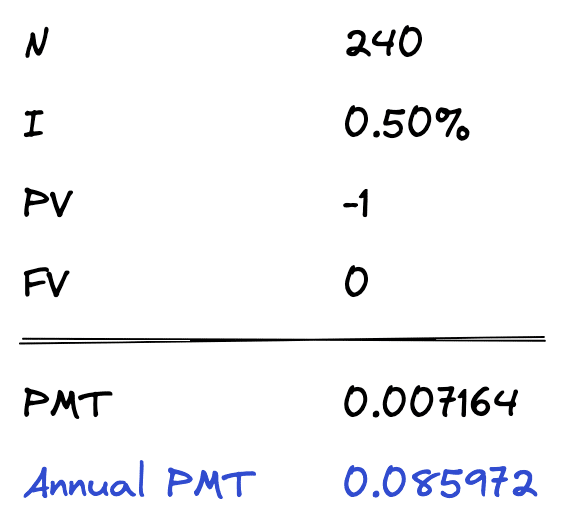
When we solve for payment, we get 0.007164. Since this is a monthly payment, we can multiply by 12 to get an annual mortgage constant of .085972. Notice this is the same 8.5972% mortgage constant we found above. So, we have two different approaches to calculate the mortgage constant that will give us the same result.
Mortgage Constant Example: Band of Investment
Once you have calculated the mortgage constant, it can be used in a variety of ways. Let’s take a look at a mortgage constant example that uses the band of investment approach to calculate the cap rate, which is commonly used by appraisers.

The band of investment method is a popular appraisal approach to deriving a market-based cap rate. It’s frequently used by appraisers to support a market cap rate used in the income approach to valuation. The band of investment method is simply a weighted average of the returns to both debt and equity. These returns can be found by surveying lenders to find out their typical loan terms for a particular property, and also surveying investors to find out their required cash on cash returns for a particular property. Let’s take a look at how the mortgage constant is used with the band of investment.
Suppose we want to find an appropriate cap rate to value an office property in Orlando, FL. First, we can call around to several lenders in the area and ask them what their current loan terms are for this kind of property. If lenders are currently underwriting office properties at a 75% loan to value, with a 25-year amortization, and a 5% interest rate, then we can calculate the mortgage constant using one of the methods above. When we do this, the resulting annual mortgage constant is 0.07015.
Next, we can survey local investors to see what their required cash on cash return would be to invest in a property like ours. Suppose our investor survey reveals an average 11% cash on cash return requirement. Now we can use these debt and equity returns to estimate a market-based cap rate using the band of investment method.
To accomplish this, we simply take a weighted average of the two rates of returns to get 8%. This is found by taking the mortgage constant times the LTV ratio, then adding this result to the cash on cash return times 1 minus the LTV ratio: (7.015% x .75) + (11% x .25) = 8.01%, which would most likely be rounded down to 8%.
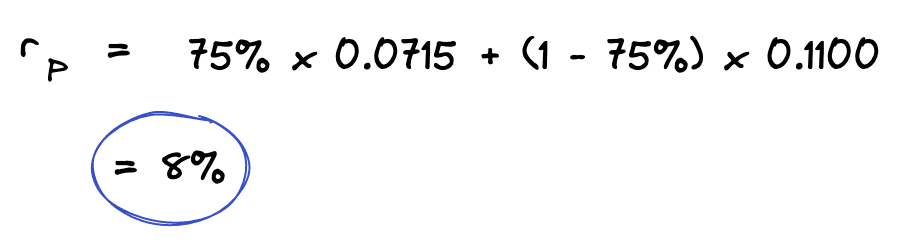
Of course, there are pros and cons to using the band of investment method to estimate a market-based cap rate, but it’s frequently used in the commercial real estate industry and the mortgage constant is a critical component.
Conclusion
The mortgage constant, sometimes called the loan constant, the debt constant, or the mortgage capitalization rate, is a commonly used calculation in real estate finance. In this article, we defined the mortgage constant, discussed two common approaches to calculating the mortgage constant, and then we showed how the mortgage constant is used with the band of investment approach to calculating the cap rate.
