Interpreting interest rates is not always as straightforward as it seems, and getting it right can have important implications for all parties involved in a commercial real estate transaction. Interest rates can take two forms: nominal interest rates and effective interest rates. As a result, there can be some confusion about what a quoted interest rate actually means.
In this article, we will take a deep dive into the differences between nominal and effective rates. We’ll start with the basic intuition behind effective and nominal rates, then walk through the math step by step, with several examples along the way.
EXECUTIVE Summary
- Nominal vs. Effective Rate: The nominal rate is the stated rate without compounding; the effective rate takes compounding into account and shows the actual rate of increase over a given period.
- Why It Matters: Misunderstanding these two rates can lead to inaccurate calculations, especially in commercial real estate transactions involving loans and investment returns.
- Key Outcomes: By the end, you’ll know how to calculate both rates, convert between them, and use these concepts to compare different loan or investment scenarios accurately.
Table of Contents
- Different Ways Interest Rates Are Quoted
- What is an Effective Interest Rate?
- What is a Nominal Interest Rate?
- Effective Interest Rate Equations
- Compounding Frequency and The Effective Rate
- Equivalent Nominal Annual Rate
- What Interest Rates Quotes Mean
- How to Compare Nominal and Effective Rates
- What About the Internal Rate of Return?
- Conclusion
Different Ways Interest Rates Are Quoted
First, let’s distinguish between the terms “interest rate” and “rate of return”, which largely depends on perspective. When evaluating loans, the term interest rate is generally used. When evaluating investments, the term internal rate of return is generally used, which is also referred to as the rate of return or yield. In either case, we are dealing with interest rates and the concepts are the same, but this difference in language and perspective is something you should be aware of.
Next, let’s take a look at a few examples of how interest rates or rates of return might be quoted in practice:
- 10% per year
- 10% per year, compounded monthly
- 10%
- 10% compounded monthly
As you can see, it is sometimes hard to fully understand what is meant by a given interest rate. Let’s take a closer look at how to make sense of this.
SECTION REcAP: Different ways interest rates are quoted
- Interest rates can be quoted with varying time frames (annual, quarterly, monthly) and compounding frequencies.
- If the compounding period isn’t stated, it’s common to assume annual compounding.
- Terminology differs based on perspective: “interest rate” for loans vs. “rate of return” or “yield” for investments.
What is an Effective Interest Rate?
First of all, what is an effective interest rate? An effective interest rate takes into account the effect of compounding and gives the actual rate of increase over the stated time period.
To illustrate, let’s look at a simple example, step by step. Suppose we deposit $100 into a bank account that earns a 1.5% effective interest rate per quarter.
Since the effective quarterly rate is 1.5%, that means after one quarter our bank account will increase by $100 x 1.5%, and we’d have $101.50 in our account.
Now, what if we want to convert this effective quarterly rate to an effective annual rate? To do this, let’s see what would happen to our bank account balance at the end of the year if we reinvest each quarterly interest payment and do not make any additional withdrawals or deposits.
At the end of the first quarter, we will have $100 x 1.015, or $101.50 in our account. At the end of the second quarter, we will have $101.50 x 1.015, or $103.02 in our account. At the end of the third quarter, we will have $103.02 x 1.015, or $104.57 in our account. Finally, at the end of the fourth quarter we will have $104.57 x 1.015, or $106.14 in our account.
Notice how we are earning interest on interest during this process. This means that we are considering the effects of compounding. So, we start out with $100. We earn 1.5% per quarter, and we end up with $106.14 at the end of the year.
So, what is our effective annual interest rate? Our effective annual interest rate is the percentage change in our bank balance over the year, which is ($106.14-$100)/$100, or 6.14%.
This effective annual rate considers the effects of compounding during the year (earning interest on interest) and gives us the actual rate of increase that would occur over the stated time interval (which in this case is a year), assuming no additional deposits or withdrawals are made.
We’ll derive a more generalized effective rate formula later, but for now, focus on the intuition behind what the effective rate is doing, which is taking into account the effect of compounding over the stated time period.
Section recap: what is an effective interest rate?
- The effective interest rate includes the impact of compounding within the stated time period.
- It shows the actual percentage change in an account balance or investment over that period.
- Example: A 1.5% effective quarterly rate leads to a 6.14% effective annual rate when interest compounds quarterly.
What is a Nominal Interest Rate?
Now that we understand what an effective interest rate is, what is a nominal interest rate? A nominal interest rate expresses the total interest paid in a period, without considering the effects of compounding during the stated time interval.
When rates are quoted as nominal rates, it is customary to provide 1) a stated rate per period, and 2) the number of times interest is compounded during that period.
Let’s take a closer look at how this works.
In our example above, we deposited $100 into a bank account earning a 1.5% effective interest rate per quarter. We saw how the effective annual interest rate was 6.14% because it took into account compounding during the year. So, what would our nominal annual interest rate be in this case?
Since the nominal interest rate ignores the effect of compounding, we can find the nominal annual interest rate by simply multiplying our quarterly effective rate by four quarters in a year to get our nominal annual rate. In this case, that would be 1.5% x 4, which results in 6%.
Recall that with nominal interest rates we need to provide 1) a stated rate per period, and 2) the number of times interest is compounded during that period. So, in this case we would say that we have a nominal rate of 6% per year, compounded quarterly.
Notice how this simple conversion from a quarterly rate to an annual rate only involved multiplication, and therefore did not consider the effects of compounding. Since multiplication is just repeated addition, we are simply adding up the interest paid on the initial balance for each quarter. We use the initial balance in our interest calculations because we are not considering the effects of compounding, and therefore we are not earning interest on interest.
In other words, if we were to use our bank account example again, then at the end of the first quarter we are owed $100 x 1.015 in interest. At the end of the second quarter, we are owed another $100 x 1.015 in interest. At the end of the third quarter we are again owed $100 x 1.015, and at the end of the fourth quarter we are yet again owed $100 x 1.015. Since we are owed 1.5% on our initial balance each quarter, we can just multiply our 1.5% quarterly interest rate by 4 quarters to get 6%.
Notice that this 6% interest rate does not give us the actual increase in our bank account for the year, considering the effects of compounding. Another way to see that this 6.00% nominal annual rate does not consider compounding is to recall that in the effective annual rate exercise above (which did take into account compounding), our bank balance increased by 6.14% at the end of the year.
section recap: what is a nominal interest rate?
- The nominal rate ignores the effect of compounding during the stated period.
- Usually quoted alongside a compounding frequency (e.g., 6% nominal annual rate, compounded quarterly).
- Unlike the effective rate, it simply multiplies the rate by the number of compounding periods without adding “interest on interest.”
Effective Interest Rate Equations
Now that we have a basic intuition about effective and nominal rates, let’s formalize the relationship between effective and nominal rates and take a closer look at how the math works.
General Relationship Between Nominal Annual Rate and Effective Annual Rate
We can start by deriving a generalized equation for calculating the effective interest rate as follows. If $1 was deposited into an account that compounded interest m times per year and paid a nominal interest rate per year of r, then the effective interest rate per compounding period would be r/m. That means the total amount in the account at the end of the year would be:
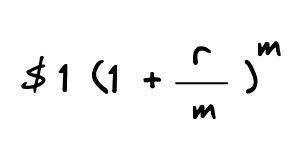
This reduces to:
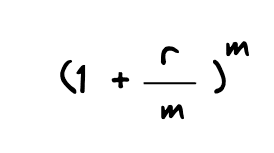
If we then deduct the $1 principal sum, then we’d get:
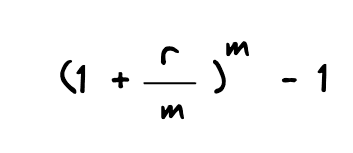
So, the effective annual rate formula is therefore:
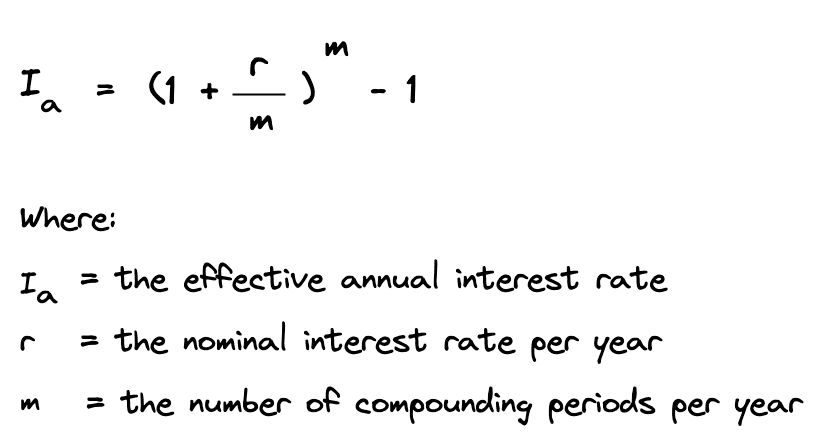
For example, recall that we saw how earning a 1.5% effective rate per quarter could be quoted as a 6% nominal annual rate, compounded quarterly. Let’s take this 6% nominal annual rate, compounded quarterly, and plug it in our formula to find the effective annual rate. To accomplish this we just need to know r, the nominal interest rate per year, and m, the number of compounding periods per year. In this case, r is 6% and m is 4. So, that gives us:
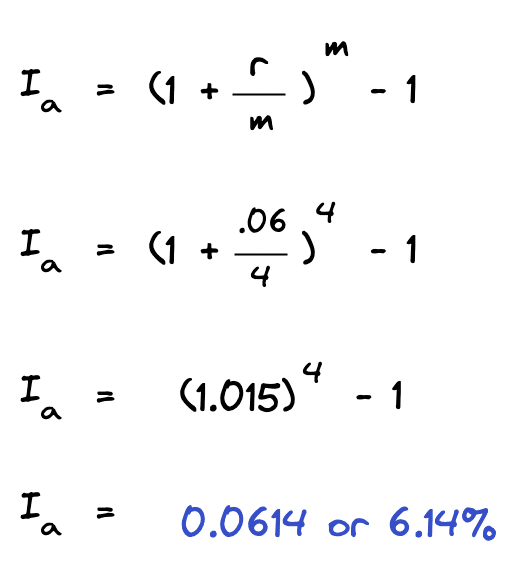
Notice this is the same result we manually calculated in the effective interest rate discussion above.
Effective Annual Rate For Any Time Period
To find the effective interest rate for a period other than yearly, you can change the time period used for the variable r and m as required. For example, the effective interest rate per month is:

For instance, suppose we had an effective rate of 1% per month, compounded daily using a 30-day month. In this case, our effective monthly rate would be 1.01%:
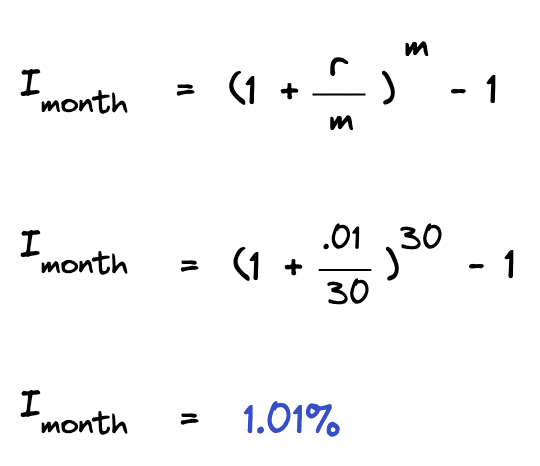
How to Convert an Effective Rate Per Compounding Period into an Effective Annual Rate
If we substitute the effective interest rate per compounding period, i = (r/m), then we can also calculate the effective annual rate as follows:

For example, if we have an effective rate of 1% per month, we would convert this to an effective annual rate as follows:

How to Convert an Effective Annual Rate into an Effective Rate Per Compounding Period
Alternatively, if we know the effective annual rate and want to find the effective interest rate per compounding period, then we can also rearrange the equation above to solve for i:
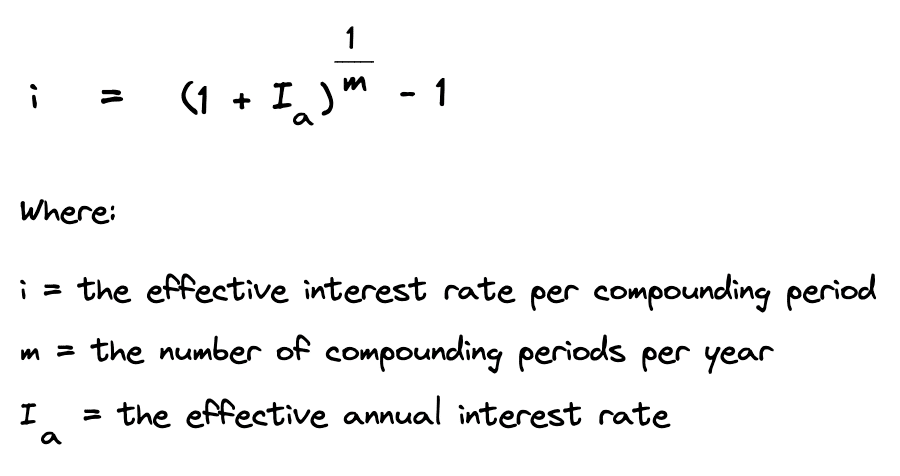
For example, if we have an effective annual rate of 12.68% we could convert this to an effective monthly rate as follows:
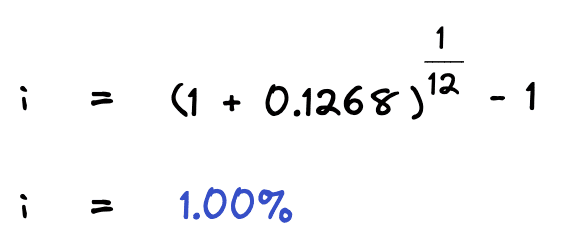
Next, let’s take a closer look at how changing the compounding frequency impacts the effective rate.
section recap: effective interest rate equations
- You can derive the effective annual rate from the nominal rate using the formula (1+r/m)^m -1
- Formulas allow conversion between different compounding frequencies and time frames.
- Knowing how to move from a nominal to an effective rate (and vice versa) is crucial for accurate comparisons
Compounding Frequency and The Effective Rate
The effective interest rate can be equal to or greater than the nominal interest rate. Let’s first take a look at the case where the effective rate equals the nominal rate. Then we will look at how the effective interest rate becomes greater than the nominal interest rate.
When the Effective Rate is Equal to the Nominal Rate
When interest compounds only once per time period, the effective interest rate and the nominal interest rate per time period will be equal to each other. Earlier, we derived the effective annual rate formula as follows:
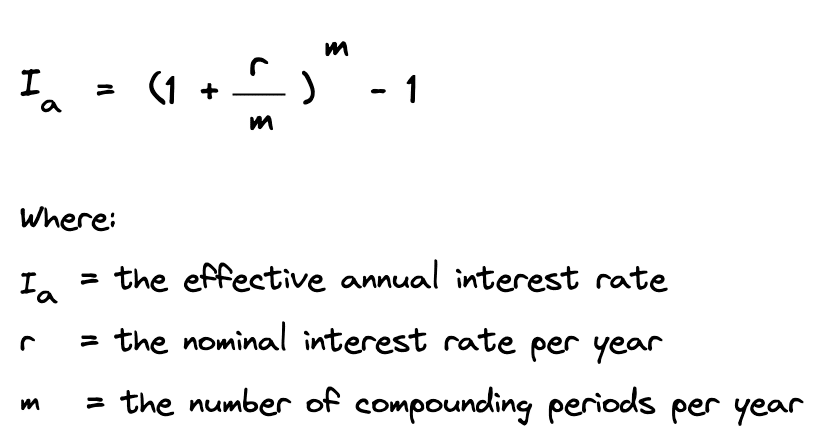
When we substitute in 1 for m, the number of compounding periods per year, we get the following:
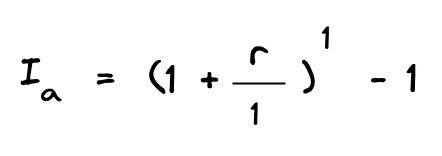
Which reduces to:
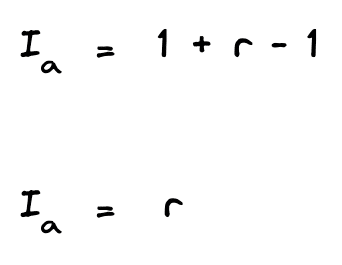
In other words, when the interest time period matches the interest compounding period, then the nominal and effective interest rates will be the same.
To see this with an example, suppose we have a nominal interest rate of 10% per year, compounded annually. In this case, the interest period is yearly, and the compounding period is also yearly. If we plug this into the formula above, then we get:
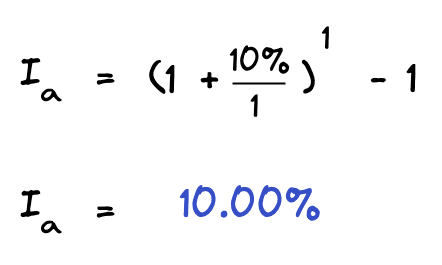
As you can see, the nominal interest rate per time period is equal to the effective interest rate per time period when compounding occurs only once per time period.
When the Effective Rate is Greater than the Nominal Rate
It is also possible for compounding to occur more frequently than once per time period. When compounding occurs more frequently than once per time period, the effective interest rate will be greater than the nominal interest rate.
For example, suppose we have a nominal rate of 10% per year, compounded monthly. If we plug this into the effective annual rate formula above then we get:
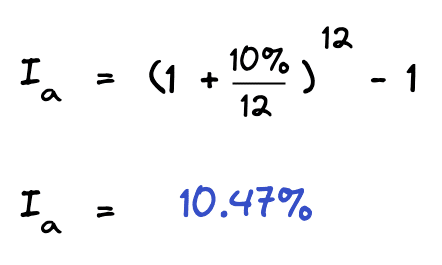
As you can see, when the interest rate is compounded more frequently than its nominal interest period, then the effective interest rate will be higher than the nominal interest rate. This idea is summarized in the table below:
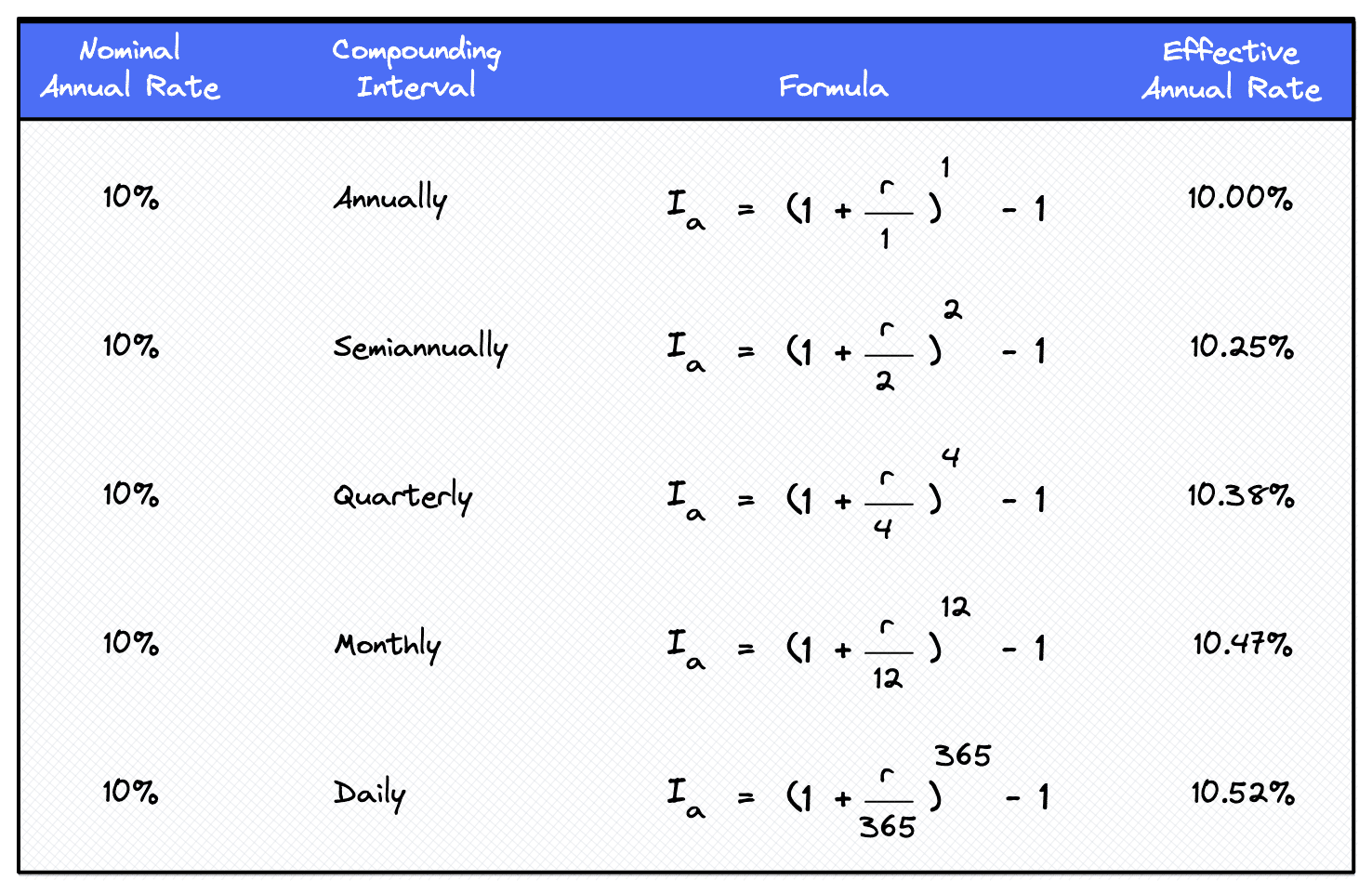
section recap: compounding frequency and the effective rate
- When interest compounds only once per period, the nominal and effective rates are the same.
- More frequent compounding (e.g., monthly vs. annually) makes the effective rate higher than the nominal rate.
- Always clarify compounding frequency to avoid confusion when comparing rates.
Equivalent Nominal Annual Rate
Sometimes we already know what the effective annual rate is, but we want to know the equivalent nominal annual rate compounded at some other frequency. For example, suppose we want to know what the nominal annual rate, compounded monthly, would have to be to provide an effective annual rate of 10%.
To figure this out, we can use the following equivalent nominal annual rate formula:
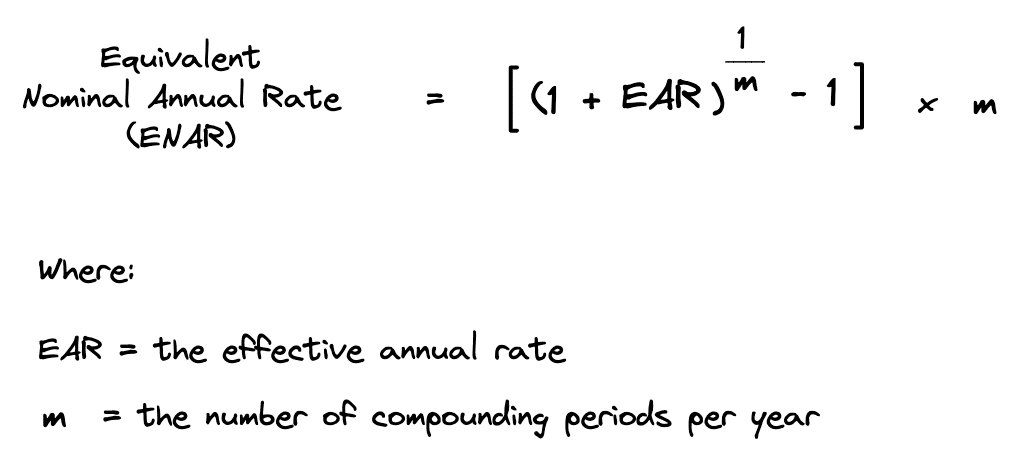
So, in the problem above, we’d have:
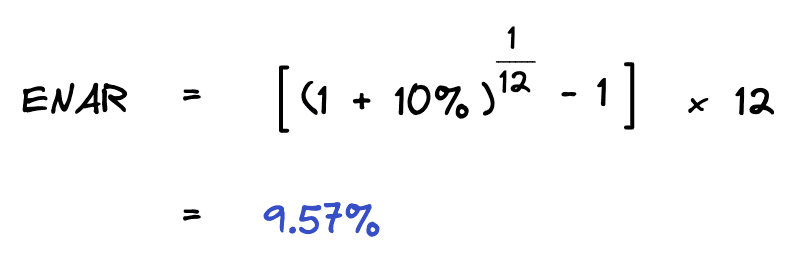
To see that this nominal rate of 9.57% per year, compounded monthly results in the same accumulated value after one year as an effective annual rate of 10%, we can solve for the future value:
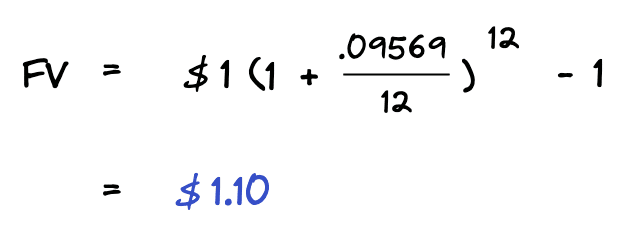
This means the effective annual rate is indeed ($1.10 – $1.00) / $1.00, or 10% because this gives the actual increase over the stated time period.
Note that any effective annual rate can be expressed in a variety of ways using different equivalent nominal annual rates that all result in the same future value after one year. This idea is summarized in the following table, using a 10% effective annual rate:
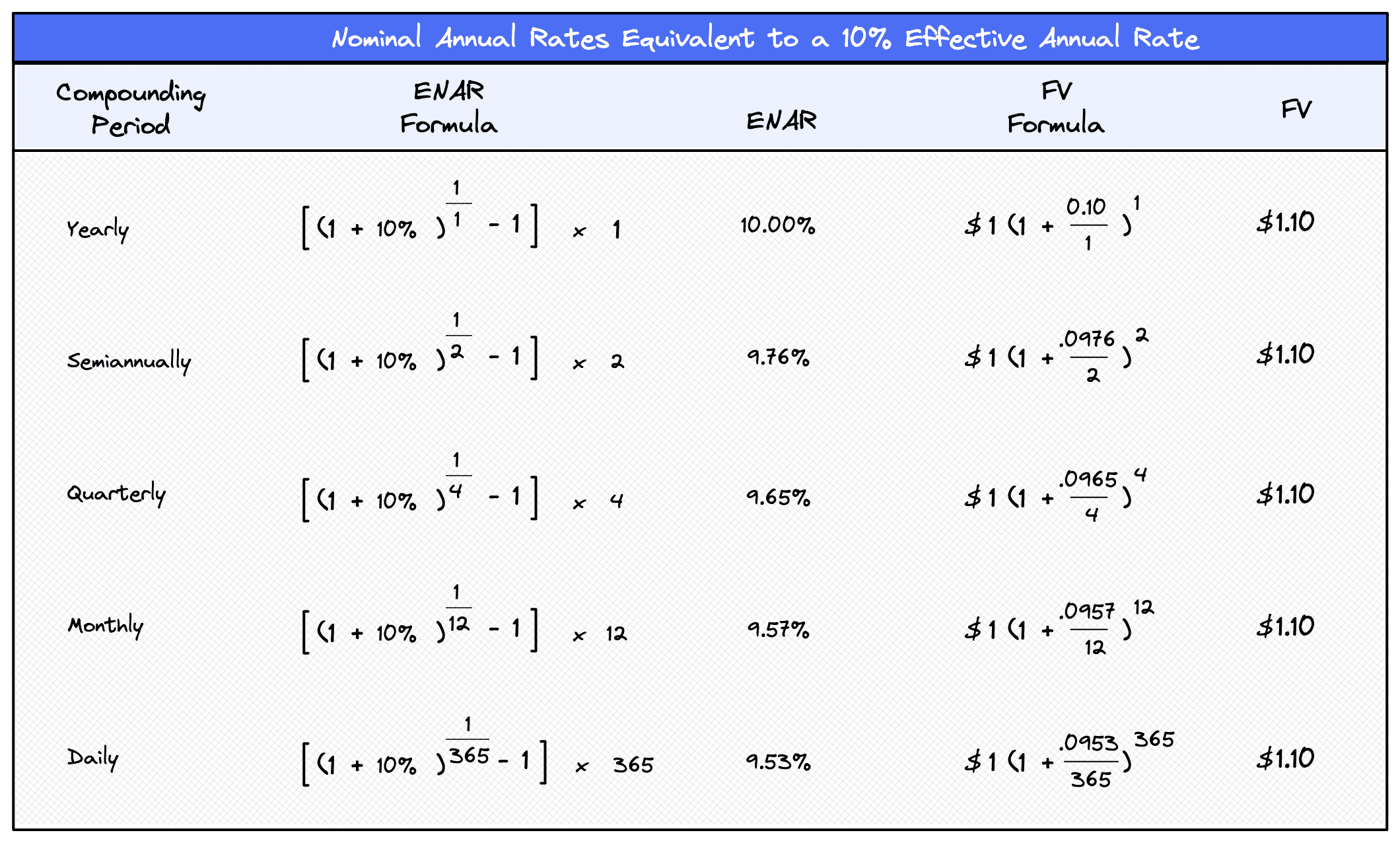
section recap: equivalent nominal rate
- Any effective annual rate can be expressed in multiple ways as an equivalent nominal annual rate with different compounding frequencies.
- Example: A 10% effective annual rate is “equivalent” to a 9.57% nominal annual rate compounded monthly.
- The key is that the future value after one year remains the same, regardless of how the nominal rate is stated.
What Interest Rates Quotes Mean
Now that we have some basic background on nominal and effective rates, let’s revisit the different ways interest rates can be quoted and what those quoted interest rates mean.
Sometimes a stated rate is described specifically as a nominal or effective rate. Other times it is not obvious. If there is ever any confusion, then it’s always best to clarify what an interest rate quote actually means with all parties involved. With that said, let’s take a closer look at common ways interest rates quotes are interpreted in practice:

The above table shows four ways interest rates or rates of return are typically quoted. These are all based on whether the time and compounding periods are provided. Again, if there is ever any doubt about what an interest rate quote means, then it’s best to clarify what is meant before different interpretations give rise to misunderstandings later. Let’s revisit the example interest rate quotes from the beginning of this article and make sense of them using the information from the table above:
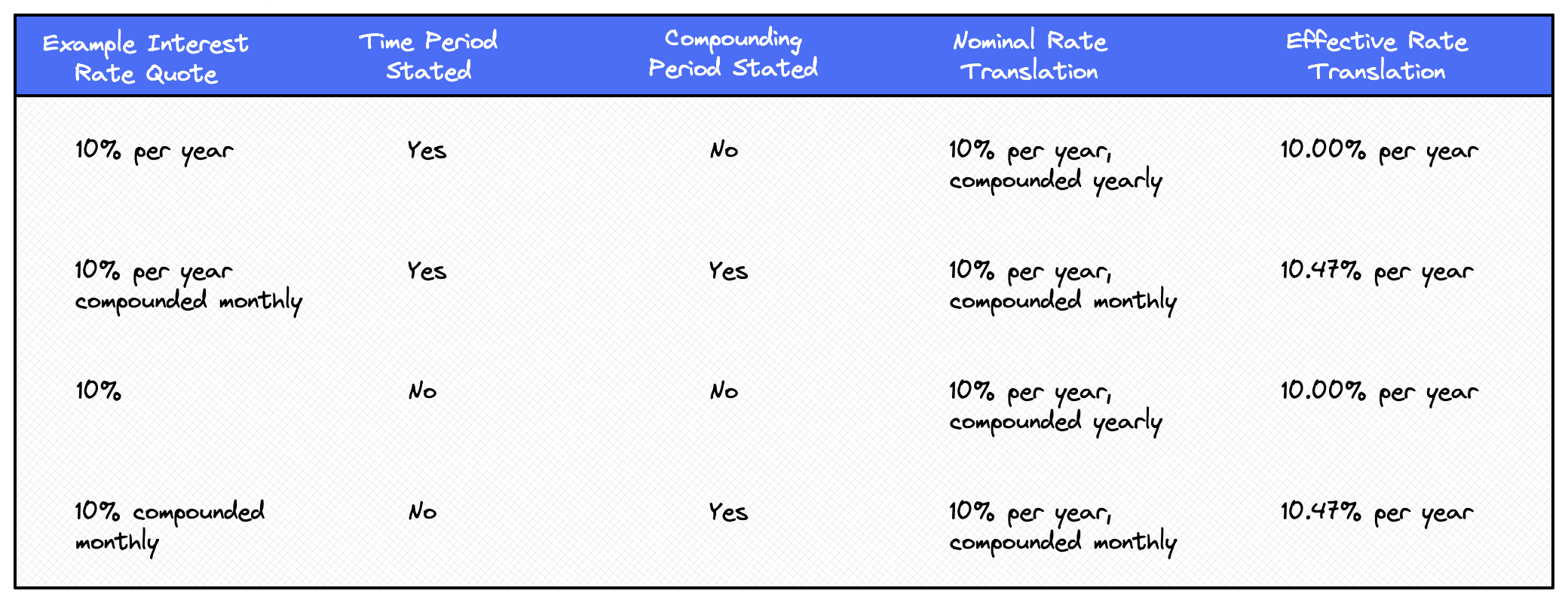
Only The Time Period Stated
When an interest rate is given with a stated time period but not with a stated compounding period, then it is convention to assume the compounding period is equal to the stated time period. For instance, a rate could be quoted as “10% per year”. In this case, it would be common to assume that the compounding period is also per year.
Recall that whenever the time period is equal to the compounding period, compounding occurs only once per period, and therefore the nominal interest rate per time period will equal the effective interest rate per time period.
That means a rate given with just a time period could be interpreted as either an effective interest rate per stated time period, or as a nominal interest rate per stated time period, where the compounding period equals the stated time period.
In our example, a given rate of 10% per year could be interpreted as a nominal rate of 10% per year, compounded yearly. It could also be interpreted as an effective rate of 10% per year.
Both The Time and Compounding Periods Stated
When an interest rate is given with a stated time period and a stated compounding period, then this can be interpreted as a nominal rate per stated time period and stated compounding period.
For example, a stated rate of 10% per year, compounded monthly is a nominal rate of 10% per year, compounded monthly. Since compounding occurs more than once per time period, the effective rate per year will be higher than the quoted interest rate of 10%. In this case, it is:
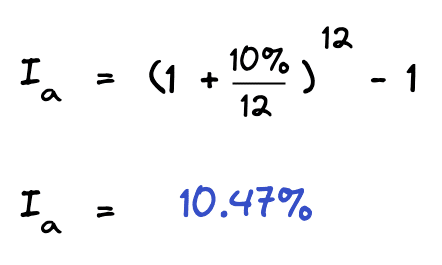
Neither The Time Nor Compounding Periods Stated
When an interest rate is given without a stated time period or a stated compounding period, then it is convention to assume 1-year for both the time and compounding periods.
For example, a rate quoted as 10% is assumed to mean a nominal interest rate of 10% per year, compounded yearly. Since the time and compounding periods are the same, the nominal and effective rate will also be the same.
Only The Compounding Period Stated
When an interest rate is given without a stated time period but with a stated compounding period, then it’s common to assume the time period is annual.
For example, a stated rate of 10% compounded monthly would be interpreted as 10% per year, compounded monthly.
Again, since compounding occurs more than once per time period, the effective rate per year will be higher than the quoted interest rate of 10%. In this case, it is:
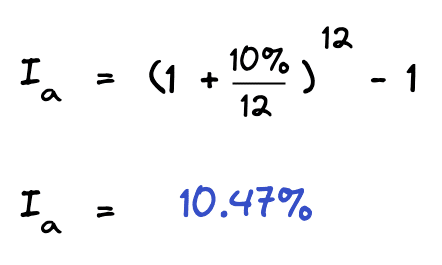
section recap: what interest rate quotes mean
- Only a Time Period Stated (e.g., “10% per year”): Assumed to be a nominal (or effective) annual rate with annual compounding.
- Both Time and Compounding Periods Stated (e.g., “10% per year, compounded monthly”): This is a nominal annual rate with monthly compounding, which generally produces a higher effective annual rate than 10%.
- Neither Time Nor Compounding Period Stated (“10%”): Commonly assumed to be 10% per year with annual compounding (nominal = effective).
- Only the Compounding Period Stated (e.g., “10% compounded monthly”): Usually taken to mean 10% per year, compounded monthly, with a higher effective annual rate than 10%.
How to Compare Nominal and Effective Rates
Only those interest rates placed on a comparable basis may be compared. Nominal interest rates can be compared if the nominal rates have the same time period and same compounding period. Otherwise, translating the nominal rates into effective rates will allow them to be compared to each other.
For example, it is easy to see that a nominal rate of 10% per year, compounded yearly is better than a nominal rate of 9% per year, compounded yearly. However, suppose the time and compounding periods were not the same. For instance, is it better to receive interest at a rate of 17% per year compounded annually or at 16% per year compounded monthly? Without translating these options into comparable rates, we can’t make a determination.
In order to make a comparison, we can translate these nominal annual rates into effective annual rates. The effective annual rate of 17% per year compounded annually is of course 17%. The effective annual rate of 16% per year compounded monthly is 17.22%:
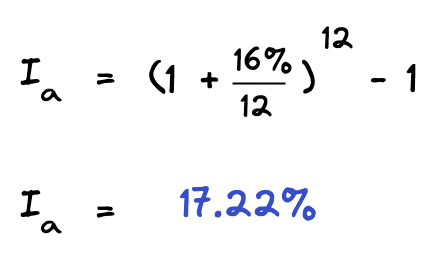
So, all else equal, the option that yields a 17.22% effective annual rate is better.
However, in the context of investment analysis, it is worth noting that all else is not always equal. For example, while one investment option may provide a better effective annual rate, there may be different equity requirements, risks, holding periods, ability to reinvest cash flows, etc. These are all considerations that should be taken into account when evaluating investment alternatives. For example, consider the following three investment options:

Notice how the investment with the highest profit has the lowest effective annual rate and the investment with the highest effective annual rate has the lowest profit. This doesn’t mean that the effective annual rate is useless. Rather, it means that there is no silver bullet when it comes to investment analysis and all relevant factors should be considered.
section recap: comparing nominal and effective rates
- Translate rates to a common basis (usually effective annual rate) before comparing.
- Example: 17% nominal annual (compounded annually) vs. 16% nominal annual (compounded monthly) → the second option actually yields 17.22% effective annual.
- Consider all investment factors (risk, holding period, reinvestment ability) in addition to rate calculations.
What About the Internal Rate of Return?
The textbook definition of the internal rate of return is that it is the interest rate that causes the net present value to equal zero. Let’s take a closer look at what this means.
Another way of defining the internal rate of return is that it is the interest rate charged on the unpaid balance of a loan. Alternatively, from the perspective of an investment rather than a loan, the internal rate of return is the interest rate earned on the unrecovered investment balance.
In the case of a loan, the interest rate is an effective interest rate per period applied to the outstanding balance of the loan in each period. For example, suppose we have a $100,000 loan amortized over 5 months at a 1% effective interest rate per month. Note, this would usually be quoted by a lender as a nominal rate of 12% per year, compounded monthly.
First we can calculate the loan payments since we are given the present value of $100,000, a 1% effective interest rate per month, the total number of periods of 5 months, and we know the future value is 0. This results in a loan payment of $20,604. Here’s a simple amortization schedule for this loan:

We start with a 100,000 loan balance in month 1. The effective interest rate of 1% is applied to this beginning of period balance to determine the $1,000 interest amount owed this month. Our payment amount is much higher at $20,604, so we deduct the $1,000 interest from our payment and are left with $19,604, which is used to pay down the principal balance of our loan. This process continues each month until we are left with a $0 balance at the end of month 5.
As illustrated by the amortization table above, the interest rate is an effective interest rate per period applied to the outstanding loan balance at the beginning of the period. Since compounding occurs only once per period, the effective interest rate of 1% per month is the same as a nominal interest rate of 1% per month, compounded monthly.
In the case of an investment, we know what the payments or cash flows are, and we need to solve for the interest rate by calculating an internal rate of return. However, in either case, the interest rate is still just the interest paid on the unrecovered balance in each period.
For example, suppose we have an opportunity that requires a $100,000 upfront investment, but will produce cash flow of $20,604 each month for 5 months. This is the same as our loan example above, except this time we are given the cash flows, and we need to solve for the interest rate by calculating an internal rate of return:
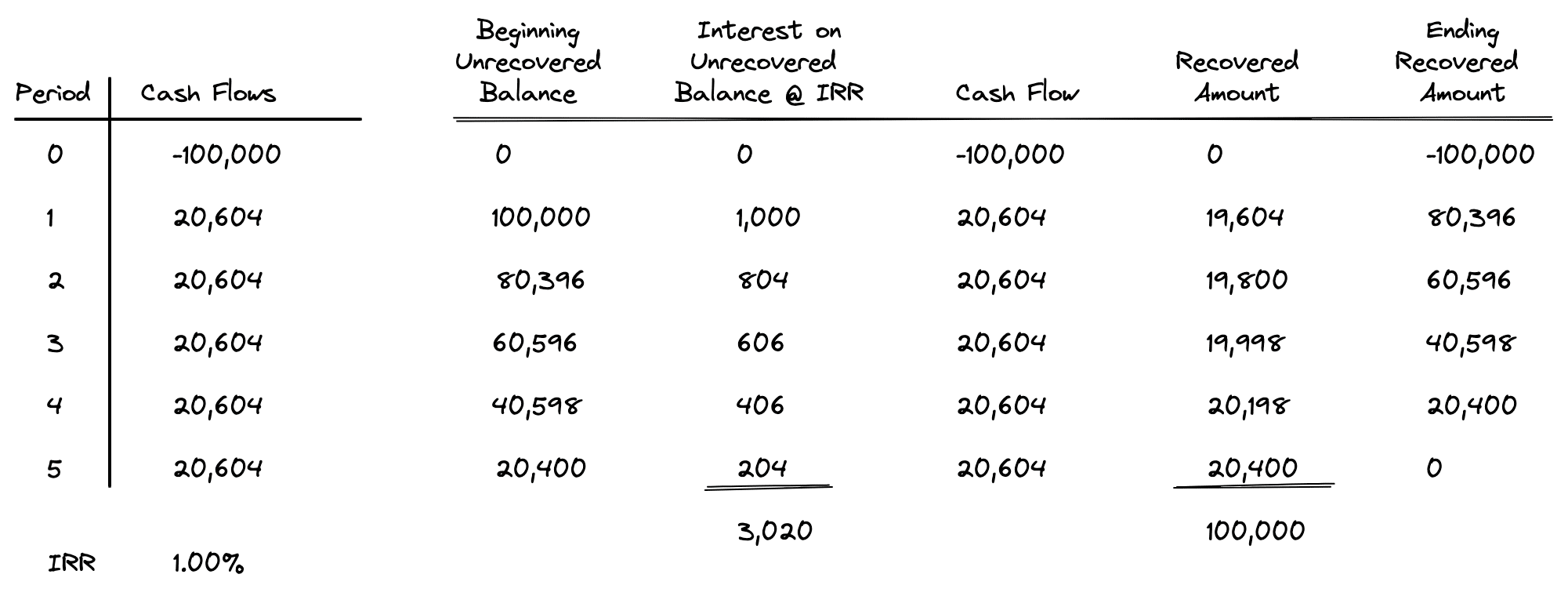
The internal rate of return on these cash flows is 1.00% per month, just like the interest rate in our loan example. We can also see that this internal rate of return is still just the interest paid on the unrecovered investment balance each period.
In month 1 the unrecovered investment balance is $100,000. Interest is owed this month at a rate of 1% (the internal rate of return), or $1,000. Our total cash flow of $20,604 is much higher, so we use the remaining $19,604 to recoup some of our investment. At the beginning of month 2 we now have $80,396 remaining in the investment and the entire process continues until we get back our entire unrecovered investment balance.
Notice how the internal rate of return is the effective interest rate per period that gives the actual rate of increase on the unpaid balance each period. And since compounding occurs only once per period, that means the nominal interest rate per period is the same as the effective interest rate per period.
Section recap: internal rate of return (IRR)
- IRR is effectively an interest rate on the unpaid or unrecovered balance each period.
- When compounding once per period, the periodic nominal rate = periodic effective rate.
- IRR applies to both loans (interest on outstanding balance) and investments (return on unrecovered capital).
Conclusion
In this article, we took a deep dive into nominal and effective interest rates. We defined the effective interest rate and nominal interest rate, derived a generalized formula for finding the effective interest rate, and then walked through several examples. We discussed what happens when the compounding frequency increases, how to express any effective rate as a variety of nominal rates, how to compare nominal and effective rates, and finally, how to interpret the internal rate of return.
final takeaways
- Nominal vs. Effective: Nominal rates are simpler to quote but can be misleading; effective rates reflect the true impact of compounding.
- Comparisons Matter: Always convert different quotes to the same basis (effective rates) before deciding on a loan or investment.
- IRR Perspective: For investors and lenders, the IRR is essentially the effective periodic rate applied to the balance owed.
- Next Step: Use these formulas to compare your financing options, investment returns, or any scenario where interest rates or yields come into play.
